Defying condensation

Particles that obey Bose statistics undergo a fascinating transition to a quantum coherent phase called Bose Einstein condensate. All particles find themselves in the lowest possible single particle state, where they coherently populate the same wave-function. Proposed in 1924 by external page Satyendra Nath Bose, this "new" quantum state was only observed in 1995. These days, the BECs, as we call these condensates, form the basis of a very broad research field and are used for metrology applications such as the most price clocks to date.

Now that we know, that the formation of a BEC is a very generic scenario, one can ask the question what happens if one prevents bosons form doing so. It turns out, that some of the most intriguing phases of matter emerge once bosons are obstructed from going their natural path. However, it is not so clear what can stand in their way.

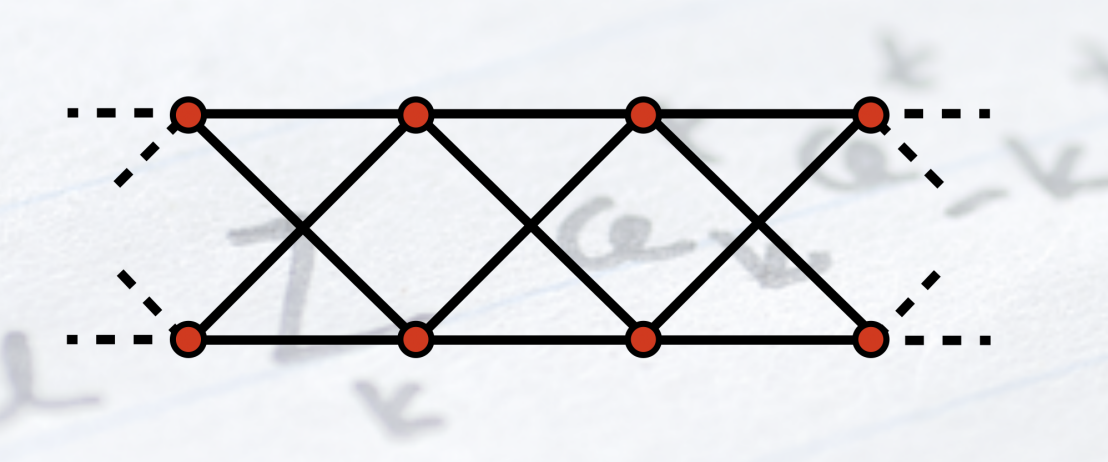
Going back to the statement "all particles find themselves in the lowest possible single particle state", an interesting strategy presents itself. There are lattices on which the Bloch bands, i.e., the single particle spectrum, is flat. With flat, we mean that there are an extensive number of states at the same energy, typically one for each point in the Brillouine zone of allowed wave-vectors. Once we have such a degenerate manifold of states, it is unclear in which state the bosons should condense. And indeed, they don't!

We explored the quantum phases emerging for bosons on the 2D kagome lattice. Depending on the density of particles, we find disordered phases of phases cohrerent "benzen-rings", valence-bond-solids at rational fillings and supersolid phases at intermediate densities. On 1D lattices with flat Bloch bands, we observe a plethora of quantum phases that are typically thought not to exist in low-dimensions. Maybe our most important finding overarching most of these phases is, that for interacting systems on flat bands, intinerant phases can arise despite the fact that single particles are immobile.